Psychrometry and Air Conditioning II

Concept of Psychrometry and Psychrometrics

Air comprises of fixed gases principally, nitrogen and oxygen with an admixture of water vapour in varying amounts. In atmospheric air water is always present and its relative weight averages less than 1% of the weight of atmospheric air in temperate climates and less than 3% by weight under the most extreme natural climatic conditions, it is nevertheless one of most important factors in human comfort and has significant effects on many materials. Its effect on human activities is in fact altogether disproportionate to its relative weights.

The art of measuring the moisture content of air is termed Psychrometry. The  science  which investigates the thermal properties of moist air, considers the measurement and control of the moisture content of air, and studies the effect of atmospheric moisture on material and human comfort may properly be termed ―psychrometrics’‘.

DEFINITIONS

Some of the more important definitions are given below:

1. Dry The international joint committee on Psychrometric Data has adopted the following exact composition of air expressed in mole fractions (Volumetric) Oxygen 0.2095, Nitrogen0.7809, Argon 0.0093, Carbon dioxide 0.0003. Traces of rare gases are neglected. Molecular weight of air for all air conditioning calculations will be taken as 28.97. Hence the gas constant,

Dry air is never found in practice. Air always contains some moisture. Hence the common designation ―air‖ usually means moist air. The term ‗dry air‘ is used to indicate the water free contents of air having any degree of moisture.

2. Saturated Moist air is said to be saturated when its condition is such that it can co- exist in natural equilibrium with an associated condensed moisture phase presenting a flat surface to it. For a given temperature, a given quantity of air can be saturated with a fixed quantity of moisture.  At  higher  temperatures, it  requires  a  larger  quantity of moisture  to saturate it. At saturation, vapour pressure of moisture in air corresponds to  the saturation pressure given in steam tables corresponding to the given temperature of air.
3. Dry-bulb temperature (DBT). It is the temperature of air as registered by an ordinary thermometer (tdb).
4. Wet-bulb temperature (WBT). It is the temperature registered by a thermometer when the bulb is covered by a wetted wick and is exposed to a current of rapidly moving air (twb).

5. Adiabatic saturation It is the temperature at which the water or ice can saturate air by evaporating adiabatically into it. It is numerically equivalent  to  the measured wet bulb temperature (as corrected, if necessary for radiation and conduction) (tdb– twb).
6. Wet bulb It is the difference between dry-bulb and wet bulb temperatures.
7. Dew point temperature (DPT). It is the temperature to which air must be cooled a tconstant pressure in order to cause condensation of any of its water It is equal to steam table saturation temperature corresponding to the actual partial pressure of  water vapour in the air (tdp).
8. Dew point    It  is  the  difference  between  the  dry  bulb  and  dew  point temperatures (tdb – tdp).

9. Specific humidity (Humidity ratio). It is the ratio of the mass of water vapour per unit mass of dry air in the mixture of vapour and air, it is generally expressed as grams of water per kg of dry For a given barometric pressure it is a function of dew point temperature alone.
10. Relative humidity(RH), (฀). It is the ratio of the partial pressure of water vapour in the mixture to the saturated partial pressure at the dry bulb temperature, expressed as
11. Sensible It is the heat that changes the temperature of a substance when added to or abstracted from it.
12. Latent It is the heat that does not affect the temperature but changes the state of substance when added to or abstracted from it.
13. It is the combination energy which represents the sum of internal and flow energy in a steady flow process. It is determined from an arbitrary datum point for the air mixture and is expressed as kJ per kg of dry air (h).

Note. When air is saturated DBT, WBT, DPT are equal.

 

Psychrometric Relations Pressure

Dalton‘s law of partial pressure is employed to determine the pressure of a mixture of gases. This law states that the total pressure of a mixture of gases is equal to the sum of partial pressures which the component gases would exert if each existed alone  in  the  mixture volume at the mixture temperature. Precise measurements made during the last few years indicate that this law as well as Boyle‘s and Charle‘s laws are only approximately correct. Modern tables of atmospheric air properties are based on the correct versions. For calculating partial pressure of water vapour in the air many equations have been proposed, probably Dr. Carrier‘s equation is most widely used.

 

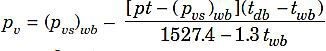
where

pv = Partial pressure of water vapour,

pvs= Partial pressure of water vapour when air is fully saturated, pt = Total pressure of moist air,

tdb= Dry bulb temperature (ºC), and twb= Wet bulb temperature (ºC).

Specific humidity W:

 

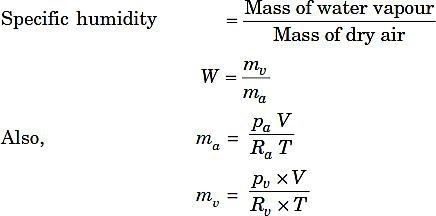
Where,

pa= Partial pressure of dry air,

pv= Partial pressure of water vapour, V= Volume of mixture,

 

 

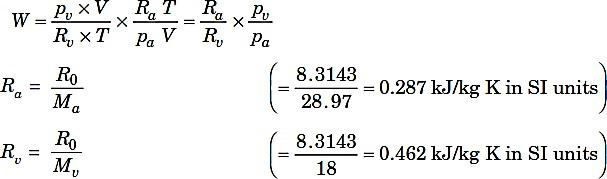
Where

R0 = Universal gas constant,

Ma= Molecular weight of air, and

Mv = Molecular weight of water vapour.

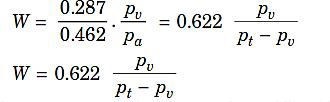
The masses of air and water vapour in terms of specific volumes are given by expression as

 

Where

va= Specific volume of dry air, and vv= Specific volume of water vapour.

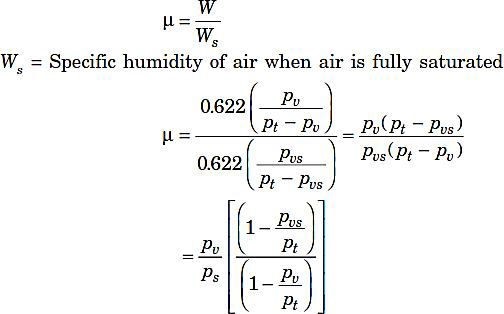
Degree of saturation (µ):

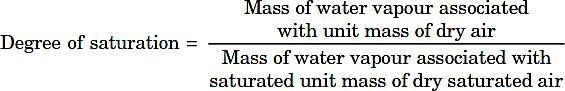
Where

pvs = Partial pressure of water vapour when air is fully saturated (pvscan be calculated from steam tables corresponding to the dry bulb temperature of the air).

Relative humidity (RH)฀ :

 

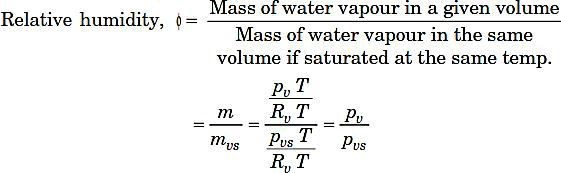
Note 1. Relative humidity as compared to specific humidity plays a vital role in comfort air- conditioning and industrial air-conditioning. Relative humidity signifies the absorption capacity of air. If initial relative humidity of air is less it will absorb more moisture.

2. W, µ and ɸ cannot be conveniently measured as they require measurement of pvand pvs. The value of pv can be obtained from the measurement of the wet bulb temperature and the value of pvscan be calculated from steam tables corresponding to given air temperature.

 

Enthalpy of moist air

It is the sum of enthalpy of dry air and enthalpy of water vapour associated with dry air. It is expressed in kJ/kg of dry air.

Where C pm= (Cp + C psW) is the specific heat of humid air or humid specific heat.

The value of C pmis taken as 1.021 kJ/kg dry air per K. It is the heat capacity of (1 + W) kg of moisture per kg of dry air.

H vapour = h g at dry bulb temperature. So,

However, a better approximation is given by the following relationship:

Where tdb is dry bulb temperature in ºC, and the datum state is liquid water at 0ºC.

 

PSYCHROMETRIC CHARTS

The psychrometric charts are prepared to represent graphically all the necessary moist air properties used for air conditioning calculations. The values are based on actual measurements verified for thermodynamic consistency.

For psychrometric charts the most convenient co-ordinates are dry bulb temperature of air vapour mixture as the abcissa and moisture content (kg/kg of dry air) or water vapour pressure as the ordinate. Depending upon whether the humidity contents are abcissa or ordinate with temperature co-ordinate, the charts are generally classified as Mollier chart and Carrier chart. Carrier chart having t dbas the abcissa and W as the ordinate finds a wide application. The chart is constructed as under:

1. The dry bulb temperature (ºC) of unit mass of dry air for different humidity contents or humidity ratios are indicated by vertical lines drawn parallel to the
2. The mass of water vapour in kg (or grams) per kg of dry air is drawn parallel to the abcissa for different values of dry bulb It is the major vertical scale of the chart.
3. Pressure of water vapour in mm of mercury is shown in the scale at left and is the absolute pressure of
4. Dew point temperatures are temperatures corresponding to the boiling points of water at low pressures of water vapour and are shown in the scale on the upper curved The dew points for

different low pressures are read on diagonal co-ordinates.

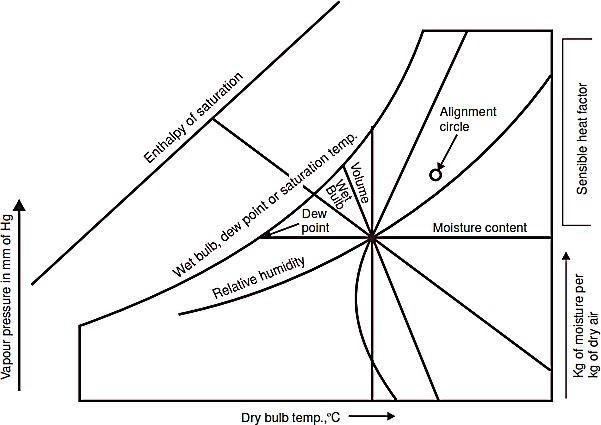
Fig.a. Skeleton psychrometric chart.

5. Constant relative humidity lines in per cent are indicated by marking off vertical distances between the saturation line or the upper curved line and the base of the The relative humidity curve depicts quantity (kg) of moisture actually present in the air as a percentage of the total amount possible at various dry bulb temperatures and masses of vapour.
6. Enthalpy or total heat at saturation temperature in kJ/kg of dry air is shown by a diagonal system of co-ordinates. The scale on the diagonal line is separate from the body of the chart and is indicated above the saturation
7. Wet bulb temperatures are shown on the diagonal co-ordinates coinciding with heat The scale of wet bulb temperatures is shown on the saturation curve. The diagonals run downwards to the right at an angle of 30º to the horizontal.
8. The volume of air vapour mixture per kg of dry air (specific volume) is also indicated bya set of

 

diagonal co-ordinates but at an angle of 60º with the horizontal.

The other properties of air vapour mixtures can be determined by using formulae (already discussed).In relation to the psychrometric chart, these terms can quickly indicate many things about the condition of air, for example:

1. If dry bulb and wet bulb temperatures are known, the relative humidity can be read from the
2. If the dry bulb and relative humidity are known, the wet bulb temperature can be deter-
3. If wet bulb temperature and relative humidity are known, the dry bulb temperature can be
4. If wet bulb and dry bulb temperatures are known, the dew point can be
5. If wet bulb and relative humidity are known, dew point can be read from the
6. If dry-bulb and relative humidity are known, dew point can be
7. The quantity (kg) of moisture in air can be determined from any of the following combinations:

• Dry bulb temperature and relative humidity;
• Dry bulb temperature and dew point;
• Wet bulb temperature and relative humidity;
• Wet bulb temperature and dew point temperature;
• Dry bulb temperature and wet bulb temperature; and
• Dew point temperature

Figs. a an b show the skeleton psychrometric chart and lines on carrier chart respectively.

 

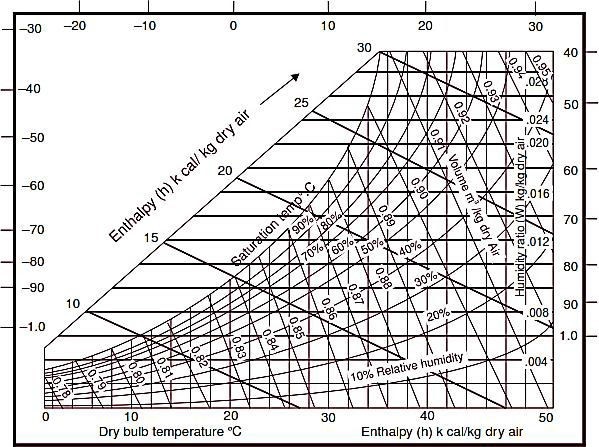
Fig b..Carrier chart.

PSYCHROMETRIC PROCESSES

In order to condition air to the conditions of human comfort or of the optimum control of an industrial process required, certain processes are to be carried out on the outside air available. The processes affecting the psychrometric properties of air are called psychrometric processes. These processes involve mixing of air streams, heating, cooling, humidifying, dehumidifying, adiabatic saturation and mostly the combinations of these. The important psychrometric processes are enumerated and explained in the following text:

4. Mixing of air streams
5. Sensible heating
6. Sensible cooling
7. Cooling and dehumidification
8. Cooling and humidification
9. Heating and dehumidification
10. Heating and

Mixing of Air Streams

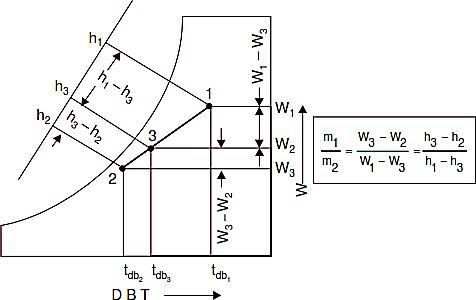
Refer Figs. C and D. Mixing of several air streams is the process which is very frequentlyused in air conditioning. This mixing normally takes place without the addition or rejection of either heat or moisture, i.e., adiabatically and at constant total  moisture content. Thus we canwrite the following equations :

 

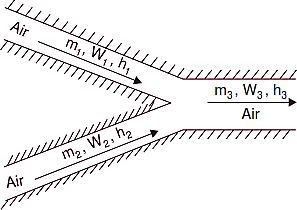
Fig. C. Mixing of air streams.

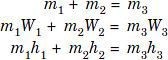
Fig D

Rearranging of last two equations gives the following:

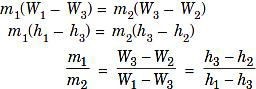
Where,

m = Mass of dry air at particular state points W= Specific humidity at particular state points h = Enthalpy at particular state points

On the psychrometric chart, the specific humidity and enthalpy scales are linear, ignoring enthalpy deviations. Therefore, the final state 3 lies on a straight line connecting the initial states of the two streams before mixing, and the final state 3 divides this line into two parts that are in the same ratio as were the two masses of air before mixing. If the air quantities are known in volume instead of

mass units, it is generally sufficiently accurate to units of m3 or m3/min. in the mixing equations.

The inaccuracy introduced is due to the difference in specific volume at two initial states. This difference in densities is small for most of the comfort air conditioning problems.

 

Sensible Heating

When air passes over a dry surface which is at a temperature greater than its (air) dry bulb temperature, it undergoes sensible heating. Thus the heating can be achieved by passing the air over heating coil like electric resistance heating coils or steam coils. During such a process, the

specific humidity remains constant but the dry bulb temperature rises and approaches that of the surface. The extent to which it approaches the mean effective surface temperature of the coil is conveniently expressed in terms of the equivalent by–pass factor.

The by-pass factor (BF) for the process is defined as the ratio of the difference between the mean surface temperature of the coil and leaving air temperature to the difference between the mean surface temperature and the entering air temperature.

Thus on Fig. E, air at temperaturetdb1, passes over a heating coil with an average surface temperature tdb3and leaves at temperature tdb2

The by-pass factor is expressed as follows :

 

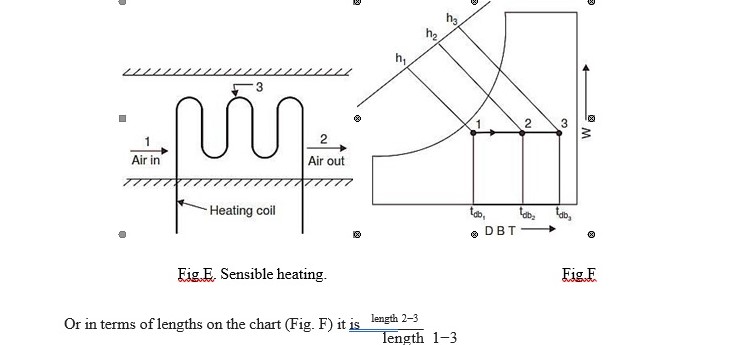
Or in terms of lengths on the chart (Fig. F) it is  length 2−3

length 1−3

The value of the by-pass factor is a function of coil design and velocity. The heat added to the air can be obtained directly from the entering and leaving enthalpies (h2-h1) or it can be obtained from the humid specific heat multiplied by the temperature difference (tdb2-tdb1).

In a complete air conditioning system the preheating and reheating of air are among the familiar examples of sensible heating.

Note. By-pass factor can be considered to represent the fraction of air which does not come into contact with coil surface.

 

Sensible Cooling

Refer Fig. G. Air undergoes sensible cooling whenever it passes over a surface that is ata temperature less than the dry bulb temperature of the air but greater than the dew point temperature. Thus sensible cooling can be achieved by passing the air over cooling coil like evaporating coil of the refrigeration cycle or secondary brine coil.

During the process, the specific humidity remains constant and dry bulb temperature decreases, approaching the mean effective surface temperature. On a psychrometric chart the process will appear as a horizontal line 1–2(Fig. H), where point 3 represents the effective surface temperature. For this process:

 

The heat removed from air can be obtained from the enthalpy difference (h1-h2) or from humid specific heat multiplied by the temperature difference(tdb1-tdb2).

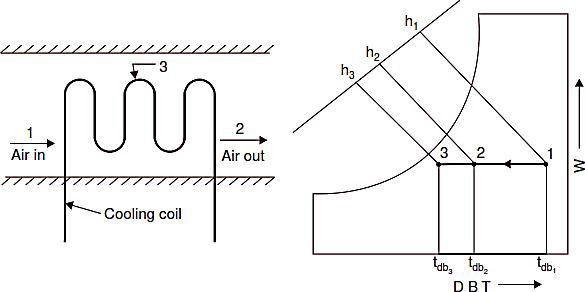
Fig.G. Sensible cooling.                                             Fig.H

 

Cooling and Dehumidification

Refer Fig. I. Whenever air is made to pass over a surface or through a spray of water that is at a temperature less than the dew point temperature of the air, condensation of some of the water vapour in air will occur simultaneously with the sensible cooling process. Any air that comes into sufficient contact with the cooling surface will be reduced in temperature to the mean surface temperature along a path such as 1-2-3 in Fig. I, with condensation and therefore dehumidification occurring between points 2 and 3. The air that does not contact the surface will be finally cooled by mixing with the portion that did, and the final state point will somewhere on the straight line connecting points 1 and 3. The actual path of air during the path will not be straight line shown but will be something similarly to the curved dashed line 1–4.

 

It will result from a continuous mixing of air which is connecting a particular part of the coil and air which is by passing it. It is convenient, however to analyse the problem with the straight line shown, and to assume that the final air state results from the mixing of air that has completely by passed the coil with air that has been cooled to the mean effective surface temperature. If there is enough contact between air and surface for all the air to come to the mean surface temperature, the

process is one of zero by pass. In any practical system, complete saturation is not obtained and final state will be a point such as 4 in Fig. I with an equivalent by pass factor equal to length 3−4 or processes involving condensation, the effective length 3−1 surface temperature, e.g tdb3i

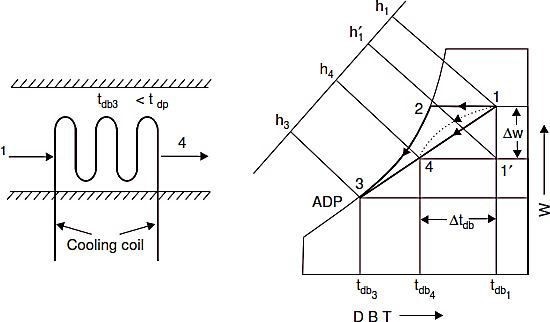
Shown in Fig. I is called apparatus dew point‘ (ADP). The final state point of air passing through a cooling and dehumidifying apparatus is in effect a mixture condition that results from mixing the fraction of the air, which is equal to the equivalent by- pass factor (BF) and is at initial state point and the remaining fraction which is equal to one minus by pass factor (1–BF) and is saturated at the apparatus dew point (ADP).

Total heat removed from the air is given by

The ratio fixes the slope of the line 1—4 on the psychrometric chart. Sensible heat factor slope lines are given on the psychrometric chart. If the initial condition and SHF are known for the given process, then the process line can be drawn through the given initial condition at a slope given by SHF on the psychrometric chart.

The capacity of the cooling coil in tonnes of refrigeration is given by,

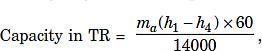
Where ma= mass of air, kg/min and h = enthalpy in kJ/kg of air.

Cooling and Humidification

If unsaturated air is passed through  a spray of continuously recirculated water, the specific humidity will increase while the dry bulb temperature decreases. This is the process of adiabatic saturation  or  evaporative  cooling.  This  process  is  one  of  constant  adiabatic-  saturation temperature and for all practical purposes, one of constant wet bulb temperature. The process is illustrated as path 1-2 on Fig. J, with wet bulb temperature of air being that of point 3, which is also equilibrium temperature of the recirculated water if there is sufficient contact between air and spray, the air will leave at a condition very close to that of point 3. The concept of equivalent by pass can be applied to this process but another term is more used to describe the performance of a humidifying apparatus. It is the ‘saturating’ or ‘humidifying efficiency’ which is defined as the ratio of dry-bulb temperature decrease to the entering wet bulb depression usually expressed as percentage. Thus, from Fig. J, the saturating efficiency is :

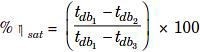
As a fraction, it is equal to one minus the by pass factor for the process. This adiabatic process, for all practical purposes, is line of constant enthalpy. The moisture added can be obtained from the increase in specific humidity.

 

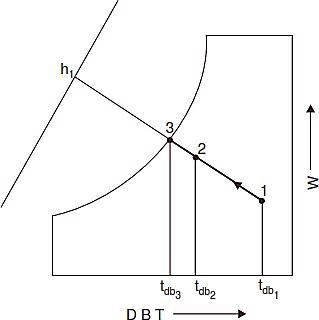
Fig J Cooling and humidification.

Heating and Dehumidification

 If air is passed over a solid absorbent surface or through a liquid absorbent spray simultaneous heating and dehumidification is accompanied. In either case the dehumidification results from adsorbent or absorbent having a lower water vapour pressure than air. Moisture is condensed out of the air, and consequently the latent heat of condensation is liberated, causing sensible heating of air. If these were the only energies involved, the process would be the inverse of the adiabatic saturation process.

There is, however, an additional energy absorbed or liberated by the active material, termed the heat of adsorption or absorption. For the solid adsorbents used commercially, such as silica gel or activated alumina, and for the more common liquid absorbents, such as solutions of organic salts or inorganic compounds like ethylene, glycol, heat is involved and results in additional sensible heating. Thus the path lies above a constant wet bulb line on the psychrometric chart such as path 1-2 in Fig. K

Heating and Humidification

If air is passed through a humidifier which has heated water sprays instead of simply recirculated spray, the air is humidified and may be heated, cooled or unchanged in temperature. In such a process the air increases in specific humidity and the enthalpy, and the dry bulb temperature will increase or decrease according to the initial temperature of the air and that of the spray. If sufficient water is supplied relative to the mass flow of air, the air will approach saturation at water temperature. Examples of such processes are shown on Fig. L

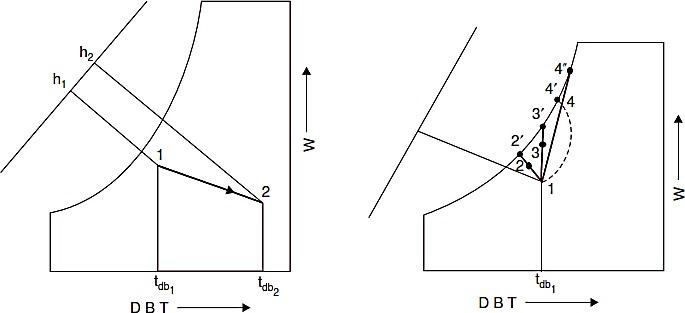
Fig. K. Heating and dehumidification.                      Fig. L. Heating and humidification.

Process 1-2 : It denotes the cases in which the temperature of the heated spray water is less than the air DBT

Process 1-3 : It denotes the cases in which the temperature is equal to the air DBT. Process 1-4 : It denotes the cases in which a spray temperature is greater than air DBT.

As in the case of adiabatic saturation, the degree to which the process approaches saturation can be expressed in terms of the by-pass factor or a saturating efficiency.

If the water rate relative to the air quantity is smaller, the water temperature will drop significantly during the process. The resultant process will be a curved line such as the dashed 1-4 where 4 represents the leaving water temperature.

Note. It is possible to accomplish heating and humidification by evaporation from an open pan of heated water, or by direct injection of heated water or steam. The latter is more common. The process line for it is of little value because the process is essentially an instantaneous mixing of steam and the air. The final state point of the air can be found, however by making a humidity and enthalpy balance for the process. The solution of such a problem usually involves cut-and-try procedure.

See also:

Vapour Absorption Refrigeration

Vapour Compression Refrigeration

Basics of Refrigeration

Air Conditioning

Psychrometry and Air conditioning